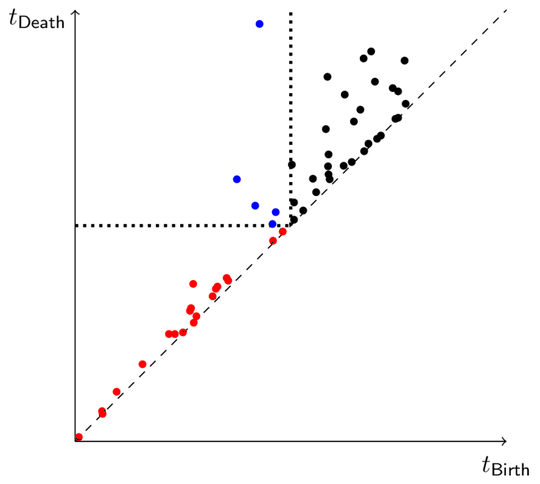
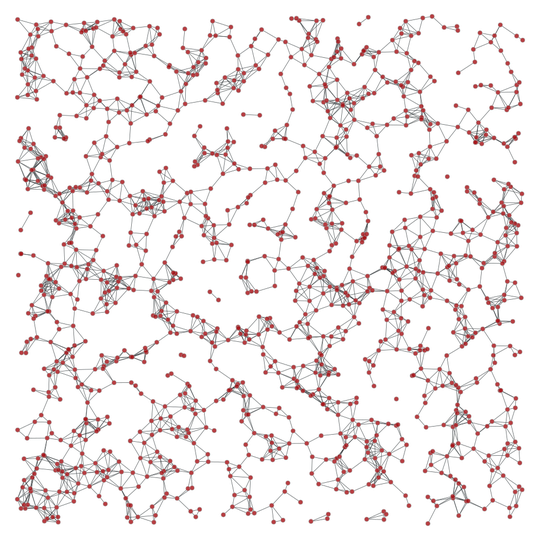
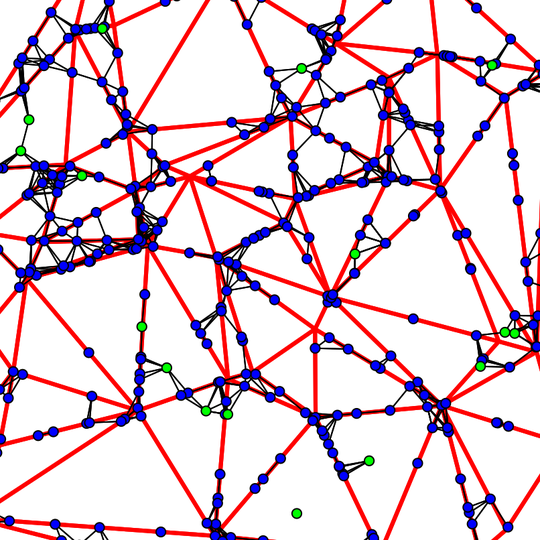
Christian Hirsch

Christian Hirsch

Associate Professor for Data Science and Statistics

Aarhus University

Christian Hirsch

I am Associate Professor for Data Science and Statistics at Aarhus University, where I am studying random networks motivated from biology and health sciences via techniques from topological data analysis and stochastic geometry. I am a member of the Stochastics group at the Department of Mathematics and an Associate Fellow of the Aarhus Institute for Advanced Studies. I am also affiliated with the AU DIGIT Centre and the AU Quantum Campus.

Before that, I was Assistant Professor at the University of Groningen and at the University of Mannheim. I was Postdoc at Aalborg University, at the LMU Munich and the WIAS Berlin. I received my PhD from Ulm University.

Open PhD and postdoc positions. I am looking for a motivated PhD student and postdoc to work with me in the domain of topological data analysis and spatial random networks. Please contact me if your are interested.

TDA summer school; August 04 - August 08, 2025 in Aarhus, Denmark. We are glad to announce the summer school Topological data analysis in stochastic geometry and image processing. Details on registration to be announced soon.

Keynote speakers:

  • Omer Bobrowski
  • Anna Gusakova
  • Anthea Monod
  • Raphaël Lachièze-Rey

Interests

  • Statistical foundations of topological data analysis
  • Large deviations theory in stochastic geometry
  • Percolation theory of spatial random networks

Education

  • PhD in Mathematics, 2014

    Ulm University

  • Diploma in Mathematics, 2010

    LMU Munich

Projects

Topological data analysis

Topological data analysis is based on an equally simple as intriguing principle. Leverage invariants from algebraic topology to gain novel insights into data. TDA is now applied in a wide variety of disciplines.

Large deviations

What is the probability that a random geometric graph in a sampling window has atypically few or atypically many edges or triangles? What are the sources leading to such rare events? These examples illustrate the core questions of large devations in geometric probability.

Spatial random networks

In the context of the Internet of Things and in 5G cellular networks, Device-to-Device (D2D) communication plays a key role. This technology aims to reduce the load on the base station by allowing users to communicate with one another, either directly or through several intermediate steps.

Research

Quickly discover relevant content by filtering publications.

Large deviations of one-hidden-layer neural networks

We study large deviations in the context of stochastic gradient descent for one-hidden-layer neural networks with quadratic loss. We …
C. Hirsch, D. Willhalm
PDF Project
Large deviations of one-hidden-layer neural networks

Large deviations for the volume of hyperbolic k-nearest neighbor balls

We prove a large deviation principle for the point process of large Poisson k-nearest neighbor balls in hyperbolic space. More …
C. Hirsch, M. Otto, T. Owada, C. Thäle
PDF Project
Large deviations for the volume of hyperbolic k-nearest neighbor balls

Lower large deviations for geometric functionals in sparse, critical and dense regimes

We prove lower large deviations for geometric functionals in sparse, critical and dense regimes. Our results are tailored for …
C. Hirsch, D. Willhalm
PDF Project
Lower large deviations for geometric functionals in sparse, critical and dense regimes

Upper large deviations for power-weighted edge lengths in spatial random networks

We study the large-volume asymptotics of the sum of power-weighted edge lengths in Poisson-based spatial random networks. In the regime …
C. Hirsch, D. Willhalm
PDF Project
Upper large deviations for power-weighted edge lengths in spatial random networks

Face and cycle percolation

We consider face and cycle percolation as models for continuum percolation based on random simplicial complexes in Euclidean space. …
C. Hirsch, D. Valesin
PDF Project
Face and cycle percolation
See all publications

Teaching

Supervision

  • Postdocs
  • PhD theses
    • N.N. Lundbye (since 02/23). Statistical foundations of TDA
    • P. Juhasz (since 11/22). TDA-based models of evolving higher-order networks
    • D. Willhalm (05/20 - 04/24). Limit theory for spatial random networks
  • MSc theses
    • L. de Jonge (07/21). Absence of WARM percolation on geometric networks
    • Y. Couzinié (09/18). Sublinearly reinforced Pólya urns on graphs of bounded degree
    • F. Rudiger (09/18). Recurrence and transience of graphs generated by point processes
    • A. Hinojosa Calleja (08/16). Interference in ad-hoc telecommunication systems in the high-density limit
    • E. Rolly (06/16). Gibbs-Masse für Trajektorien von Nachrichten in einem Kommunikationsnetzwerk
    • A. Tóbiás (04/16). Highly dense mobile communication networks with random fadings
  • BSc theses
    • K.B. Bräuner and A. Kristensen (05/24). Forudgående tidsbestilling for patienter
    • J. Borg (05/24). Markov beslutningsteori: Optimal opladning af elbiler
    • A.P. Diakovasilis and L.V. Jacobsen (05/24). Optimal ambulanceudsendelse
    • M.-C. Mociran (05/24). Optimering af blodplader oplagring
    • F. Tækker (05/24). Screening og behandling af kroniske sygdomme
    • C. Teoridis (05/24). Optimal tildeling af forespørgsler blandt tradløse sensornetværk og databaser
    • C.S. Pallesen (05/24). Forudgående patientkonsultationsplanlægning
    • H.C. Hansen (01/24). Convex hull algorithms for the triangulation/construction of alpha complexes
    • B.H. Kristensen (06/23). Asymptotic normality of tessellation - based persistent Betti numbers
    • B. Buttenschøn (06/23). A law of large numbers for wide one-layer neural networks
    • F. Brück (06/17). Percolation properties of Poisson graphs
    • L. Kriouar (07/21). Analysis of financial data with time series and persistent homology
    • H. Hong (07/21). Geometric and topological approaches to mode clustering
    • J. Langenbahn (12/19). Konvergenz des Pseudo-Marginalen MCMC Verfahren
    • H. Blocher (02/18). Poisson Matching

Contact