Abstract
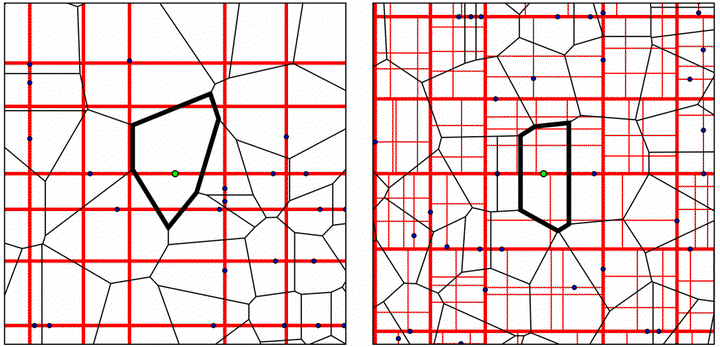
The typical cell is a key concept for stochastic-geometry based modeling in communication networks, as it provides a rigorous framework for describing properties of a serving zone associated with a component selected at random in a large network. We consider a setting where network components are located on a large street network. While earlier investigations were restricted to street systems without preferred directions, in this paper we derive the distribution of the typical cell in Manhattan-type systems characterized by a pattern of horizontal and vertical streets, designed to model real cities with a gridlike layout. We explain how the mathematical description can be turned into a simulation algorithm, provide numerical results uncovering novel effects when compared to classical isotropic networks and finally compare the results to a real street system.