A parametric copula approach for modelling shortest-path trees in telecommunication networks

Abstract

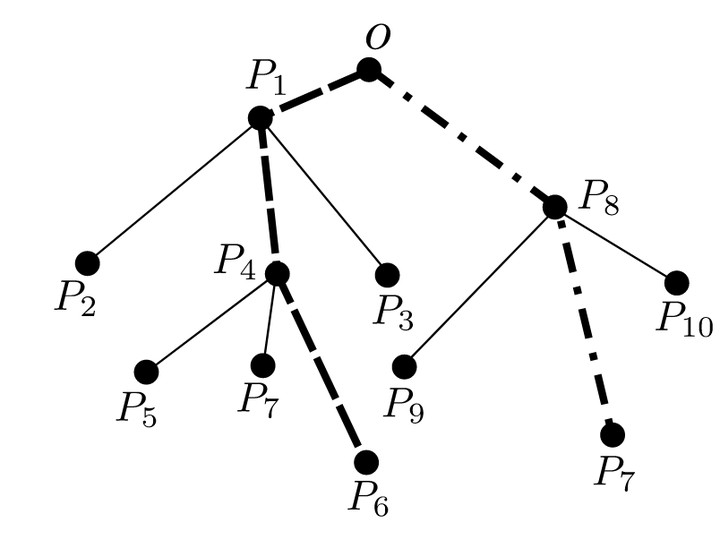
We extend the Stochastic Subscriber Line Model by the introduction of shortest-path trees which are obtained by splitting up the segment system of the typical serving zone at its crossings and endings. Due to reasons in the complex field of cost and capacity estimation in telecommunication networks, it is desirable to gain knowledge about distributional properties of the branches of these trees. The present paper shows how to obtain parametric approximation formulas for the univariate density functions of the lengths of the two main branches in shortest-path trees. Besides, we derive a joint bivariate distribution for the lengths of these branches by means of copula functions, i.e., we give a parametric composition formula of the marginals. These approximative parametric representation formulas can be used in order to prevent time consuming computer experiments.